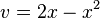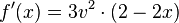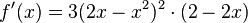Ableitungsregeln: Unterschied zwischen den Versionen
Aus Friedrich-Schiller-Gymnasium
(→Quotientenregel) |
|||
| (31 dazwischenliegende Versionen von 7 Benutzern werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| − | |||
| − | |||
== Bekannte Ableitungsregeln aus Klasse 10 == | == Bekannte Ableitungsregeln aus Klasse 10 == | ||
=== Potenzregel === | === Potenzregel === | ||
| + | Allgemeine Formel:<br /> | ||
| + | <br /> | ||
| + | <math>f(x)=x^n</math><br /> | ||
| + | <math>f'(x)=n \cdot x^{n-1} </math><br /> | ||
| + | <br /> | ||
| + | Beispiel:<br /> | ||
| + | <br /> | ||
| + | <math>f(x)=x^3+x^2-x</math><br /> | ||
| + | <math>f'(x)=3x^2+2x-1</math><br /> | ||
=== Summenregel === | === Summenregel === | ||
| + | Allgemeine Formel:<br /> | ||
| + | <br /> | ||
| + | <math>\begin{align} | ||
| + | f(x)&=u(x)+v(x) \\ | ||
| + | f'(x)&=u'(x) + v'(x) | ||
| + | \end{align}</math> | ||
=== Faktorregel === | === Faktorregel === | ||
| + | Allgemeine Formel:<br /> | ||
| + | <br /> | ||
| + | <math>f(x)=ax^n</math><br /> | ||
| + | <math>f'(x)=a \cdot n \cdot x^{n-1}</math><br /> | ||
| + | <br /> | ||
| + | Beispiel:<br /> | ||
| + | <br /> | ||
| + | <math>f(x)=2x^3+4x^2+5x</math><br /> | ||
| + | <math>f'(x)=6x^2+8x+5</math><br /> | ||
== Neue Ableitungsregeln == | == Neue Ableitungsregeln == | ||
=== Produktregel === | === Produktregel === | ||
| − | Allgemeine Formel | + | Allgemeine Formel:<br /> |
| − | <math> | + | <br /> |
| + | <math>f(x)=u(x) \cdot v(x)</math><br /> | ||
| − | === | + | <math>f'(x)=u'(x)\cdot v(x)+u(x)\cdot v'(x)</math><br /> |
| + | <br /> | ||
| + | Kurzform: <math>f'=u'v+uv'</math><br /> | ||
| + | <br /> | ||
| + | <br /> | ||
| + | Rechenbeispiel:<br /> | ||
| + | <math>f(x)=(5x^2) \cdot x^{1 \over 2}</math><br /> | ||
| + | <math>f'(x)=(10x) \cdot (x^{1 \over 2})+(5x^2) \cdot {1 \over 2}x^{-{1 \over 2}}</math><br /> | ||
| + | <math>f'(x)=10x \cdot \sqrt{x}+(5x^2) \cdot {1 \over 2}x^{-{1 \over 2}}</math> | ||
| + | |||
| + | === Quotientenregel === | ||
<math> | <math> | ||
| − | f(x)= {u(x) \over v(x)}= u'(x) | + | f(x)= {u(x)\over v(x)}</math><br /> |
| + | <math>f'(x)= {{u'(x) \cdot v(x)- u(x) \cdot v'(x)} \over (v(x))^2} | ||
</math> | </math> | ||
| + | |||
| + | Kurzform: <br /> | ||
| + | <math> f'= {{u' \cdot v- u \cdot v' } \over {v^2}} </math> | ||
| + | <br /> | ||
| + | |||
| + | Anwendungsbeispiel:<br /> | ||
| + | <math> f(x)= {{5 \cdot x^3 + 2 \cdot x^2} \over {x^2}} </math> | ||
| + | |||
| + | <math> f'= {{(15 \cdot x^2 + 4 \cdot x)\cdot x^2 }-{( 5 \cdot x^3 + 2 \cdot x^2) \cdot 2 \cdot x } \over {x^4}} </math> | ||
| + | |||
| + | <math> f'= {{(15 \cdot x^2 + 4 \cdot x^3 )} - {( 10 \cdot x^4 + 4 \cdot x^3)} \over { x^4}} </math> | ||
| + | |||
| + | <math> f'= {{ 5\cdot x^4} \over {x^4}} </math> | ||
| + | |||
| + | <math> f'= {{ 5 \cdot x^4} \cdot {1 \over{x^4}}} = {5} </math> | ||
| + | |||
| + | Quotienten lösen mit Hilfe der Produktregel: | ||
| + | Trick: Quotienten in ein Produkt umschreiben und dann die Produktregel anwenden | ||
| + | |||
| + | <math> f(x)= {{5 \cdot x^3 + 2 \cdot x^2} \over {x^2}} </math> | ||
| + | |||
| + | als Produkt: <math> f(x)= {{5 \cdot x^3 + 2 \cdot x^2} \cdot {x^-2}} </math> | ||
| + | |||
| + | <math> f'= {{(15 \cdot x^2 + 4 \cdot x) \cdot x^-2 + {( 5 \cdot x^3 + 2 \cdot x^2)} \cdot (-2 \cdot x^-3)}} </math> | ||
| + | |||
| + | <math> f'= {{x \cdot( 15 \cdot x +4 ) \over x^2} + {5 \cdot x + 2 \cdot (-2) \over x^2 } \cdot {x^2}} = {{15 \cdot x +4 \over x} - { 10 \cdot x - 4 \over x}} = {{ 5 \cdot x \over x }} = {5} </math> | ||
=== Kettenregel === | === Kettenregel === | ||
Allgemeine Formel der Kettenregel:<br /> | Allgemeine Formel der Kettenregel:<br /> | ||
<br /> | <br /> | ||
| − | <math>f | + | <math>f(x)=u(v(x))</math><br /> |
| + | <br /> | ||
| + | <math>f'(x)=u'(v) \cdot v'(x)</math><br /> | ||
| + | <br /> | ||
| + | Die Ableitung einer verketteten Funktion ist die Ableitung <br /> | ||
| + | der äußeren Funktion mal der Ableitung der inneren Funktion. | ||
| + | <br /> | ||
| + | <br /> | ||
| + | Beispiel:<br /> | ||
<br /> | <br /> | ||
<math>f(x)=(2x-x^2)^3</math><br /> | <math>f(x)=(2x-x^2)^3</math><br /> | ||
| Zeile 30: | Zeile 98: | ||
äußere Funktion: <math>u=v^3</math> | äußere Funktion: <math>u=v^3</math> | ||
<br /> | <br /> | ||
| − | innere Funktion: <math>v=2x- | + | innere Funktion: <math>v=2x-x^2</math><br /> |
<br /> | <br /> | ||
<math>f'(x)=3v^2\cdot(2-2x)</math><br /> | <math>f'(x)=3v^2\cdot(2-2x)</math><br /> | ||
| − | <math>=3(2x-x^2)^2\cdot( | + | <br /> |
| + | <math>f'(x)=3(2x-x^2)^2\cdot(2-2x)</math><br /> | ||
Aktuelle Version vom 11. Dezember 2015, 10:16 Uhr
Inhaltsverzeichnis |
Bekannte Ableitungsregeln aus Klasse 10
Potenzregel
Allgemeine Formel:
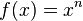
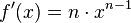
Beispiel:
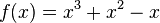
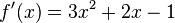
Summenregel
Allgemeine Formel:
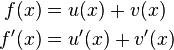
Faktorregel
Allgemeine Formel:
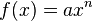
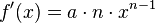
Beispiel:
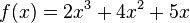
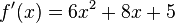
Neue Ableitungsregeln
Produktregel
Allgemeine Formel:
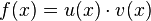
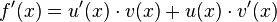
Kurzform: 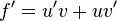
Rechenbeispiel:
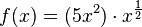
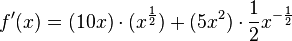
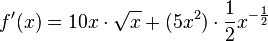
Quotientenregel
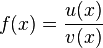
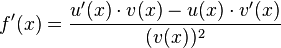
Kurzform:
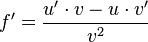
Anwendungsbeispiel:
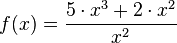
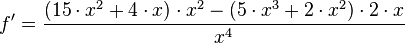
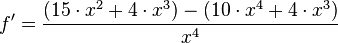
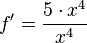
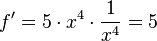
Quotienten lösen mit Hilfe der Produktregel: Trick: Quotienten in ein Produkt umschreiben und dann die Produktregel anwenden
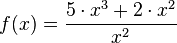
als Produkt: 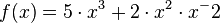
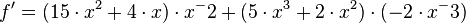

Kettenregel
Allgemeine Formel der Kettenregel:
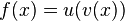
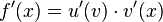
Die Ableitung einer verketteten Funktion ist die Ableitung
der äußeren Funktion mal der Ableitung der inneren Funktion.
Beispiel:
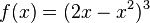
äußere Funktion: 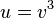
innere Funktion: